Highlights
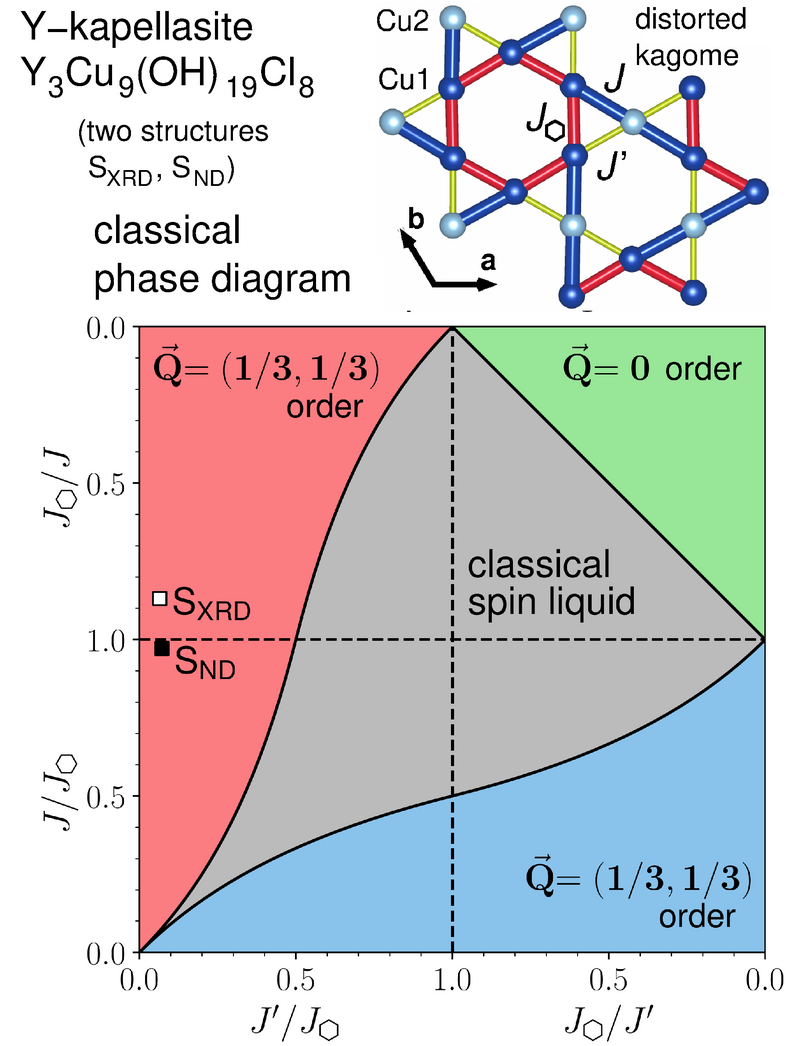
Phase diagram of a distorted kagome antiferromagnet and application to Y-kapellasite
Antiferromagnets on kagome lattices are among the most interesting materials in the field of highly frustrated magnetism. We have studied a material that has, according to our density functional theory based energy mapping, a kagome lattice with a very interesting distortion. Classical calculations arrive at a phase diagram which contains, besides three ordered phases, a large classical spin liquid phase. The material Y-kapellasite which motivated the study and led to the discovery of this phase diagram is situated in a non-collinar coplanar ordered phase, in agreement with experiment.
M. Hering, F. Ferrari, A. Razpopov, I. I. Mazin, R. Valenti, H. O. Jeschke, J. Reuther
npj Comput. Mater. 8, 10 (2022) <https://doi.org/10.1038/s41524-021-00689-0>
January 20, 2022
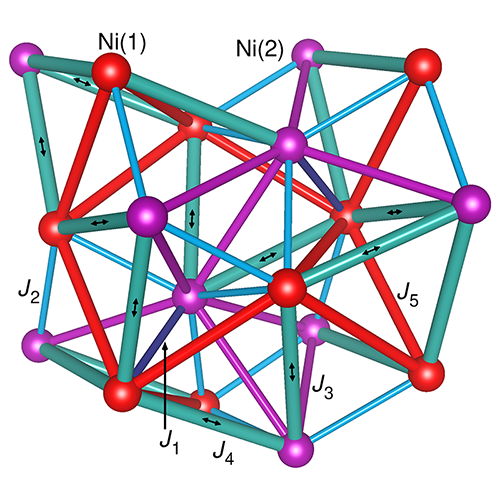
Magnetic field induced quantum spin liquid in the two coupled trillium lattices of K2Ni2(SO4)3
Quantum spin liquid candidates are usually two-dimensional materials with spin 1/2 magnetic ions. We investigated the material K2Ni2(SO4)3 with several experimental and theoretical techniques; it has Ni2+ ions with spin 1 forming a complex 3D network of two coupled trillium lattices (in the trillium lattice, each magnetic site participates in three equilateral corner-sharing triangles). Density functional theory based energy mapping identifies an intriguing model with strong antiferromagnetic couplings for both trillium lattices and for the coupling between them. Both inelastic neutron scattering and pseudo-Fermion functional renormalization group calculations show that K2Ni2(SO4)3 is a 3D material with strong quantum fluctations. Magnetic moments show a tiny static component at zero field which is suppressed by a small magnetic field, indicating that K2Ni2(SO4)3 is a magnetic field induced quantum spin liquid.
I. Živković, V. Favre, C. Salazar Mejía, H. O. Jeschke, A. Magrez, B. Dabholkar, V. Noculak, R. S. Freitas, M. Jeong, N. G. Hegde, L. Testa, P. Babkevich, Y. Su, P. Manuel, H. Luetkens, C. Baines, P. J. Baker, J. Wosnitza, O. Zaharko, Y. Iqbal, J. Reuther, H. M. Rønnow
Phys. Rev. Lett. 127, 157204 (2021) <https://doi.org/10.1103/PhysRevLett.127.157204>
September 22, 2021
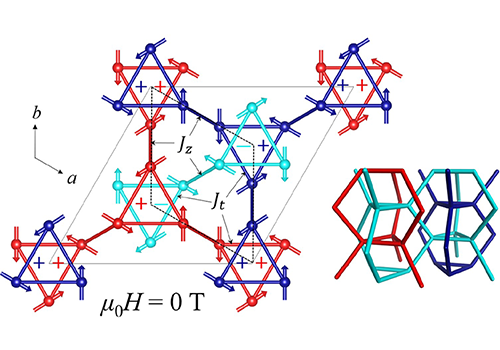
Field-tunable toroidal moment in a chiral-lattice magnet
We studied the complex magnet BaCoSiO4 with several experimental techniques and density functional theory (DFT) calculations. Inelastic neutron scattering yields an apparently very complex magnetic structure that is hard to rationalize. However, DFT based energy mapping comes up with a Heisenberg Hamiltonian that suddely explains almost all observations. The strongest couplings divide the crystal into three intertwined sublattices. In combination with small canting due to Dzyaloshinskii-Moriya interactions the sublattices turn out to have toroidal moments that can be switched by small magnetic fields due to subleading exchange interactions. This provides a mechanism to easily control the ferritoroidal and ferrotoroidal states of the system.
L. Ding, X. Xu, H. O. Jeschke,* X. Bai, E. Feng, A. S. Alemayehu, J. Kim, F. Huang, Q. Zhang, X. Ding, N. Harrison, V. Zapf, D. Khomskii, I. I. Mazin, S.-W. Cheong, H. Cao
Nat. Commun. 12, 5339 (2021) <https://doi.org/10.1038/s41467-021-25657-6>
September 09, 2021
DCore: Integrated DMFT software for correlated electrons
First-principles calculations are indispensable in researches of condensed matter physics. Using first-principles calculations based on density functional theory, the electronic structure of materials can be calculated from the chemical composition and the crystal structure. However, there is a group called strongly correlated electron systems, in which the density functional theory do not provide even qualitatively correct results. This is because the “quantum many-body effect” due to the Coulomb interaction between electrons is not considered properly. Dynamic mean field theory (DMFT) is a method that can incorporate quantum many-body effects that are lacking in density functional theory. Its demand has been increasing in recent years.
Our group is involved in the development of open-source software, DCore, that can perform dynamic mean field calculation using results of various first-principles packages. Development of a useful software is an important research for us and for condensed matter community.
H.Shinaoka, J. Otsuki, M. Kawamura, N. Takemori, K. Yoshimi
SciPost Phys. 10, 117 (2021) <https://doi.org/10.21468/SciPostPhys.10.5.117>
May 27, 2021
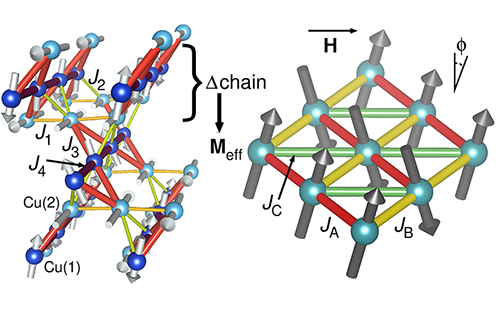
Magnetization process of atacamite: a case of weakly coupled S=1/2 sawtooth chains
The beautiful copper mineral atacamite can be found in Chile and Mexico but also in Japans Hyogo prefecture. The spin-1/2 copper ions form a highly distored three-dimensional pyrochlore lattice. Density functional theory based energy mapping, however, clarifies that only two out of four “nearest neighbour” couplings are substantial and form one-dimensional Delta chains. An apparent one-half magnetization plateau could be mistaken for the well known quantum property of Delta chains. However, it turns out that J, J’ couplings in the anisotropic Delta chain realized in atacamite are in the plateau-less J’/J limit. The suprising explanation for the magnetization that nearly stalls around 1/2 Bohr magnetons is due to a tilting of the large effective moments formed by the Delta chains in the magnetic field. Subleading exchange interactions in 3D turn out to be responsible and explain the precise magnetization dynamics.
L. Heinze, H. O. Jeschke, I. I. Mazin, A. Metavitsiadis, M. Reehuis, R. Feyerherm, J.-U. Hoffmann, M. Bartkowiak, O. Prokhnenko, A. U. B. Wolter, X. Ding, V. Zapf, C. C. Moya, F. Weickert, M. Jaime, K. C. Rule, D. Menzel, R. Valentí, W. Brenig, S. Süllow
Phys. Rev. Lett. 126, 207201 (2021) <https://doi.org/10.1103/PhysRevLett.126.207201>
May 18, 2021



